How would a sundial work on Pluto?
This sounds like a silly question because we won't be able to do the experiment any time soon. That doesn't mean that we can't answer the question, by careful reasoning, and the results are instructive.
Working out how a sundial would work on Pluto is a worthwhile exercise in celestial mechanics. It shows how days, years and seasons can behave strangely on other planets, and that even here they behave more strangely than we think.
(And the diagrams are beautiful).
We’ll warm up by seeing how sundials work here on Earth.
The apparatus
Take a thin sheet of plywood and bang a long nail through it so that it sticks out the other side. Go outdoors and put the board on the ground in the sunshine, nail side up. You have your sundial.
A sundial is a clock with no moving parts. The nail casts a shadow on the board, and the tip of the shadow tells you the time.
If you are a pedant, you may say that the sundial does have a moving part: the sun, which goes across the sky and casts a moving shadow. But then a super-pedant will come along and say that the moving part is not the sun, but the earth the sundial is sitting on. It's not a very interesting thing to argue about.
The day
Here is an example of how the sundial marks the time through the day. This sundial is at the latitude of Greenwich (51.48 degrees North), and the day is the 21st of June.
 The Sun’s shadow, Greenwich, 21 June
The Sun’s shadow, Greenwich, 21 JuneThe light blue lines go from north to south and from east to west. The nail is where the lines join. To give you a sense of scale, the radius of the circle is equal to the length of the nail.
The Sun rises in the east, so its shadow points west. When the Sun is low, the shadow is very long, so it starts from the left-hand edge of the picture. In midsummer the Sun actually rises rather north of east, so the shadow is rather south of west.
As the Sun rises in the sky its shadow gets shorter. You’ll see that at a certain point the shadow crosses the light blue circle, which means that the Sun is so high that the shadow is shorter than the nail. As the Sun rises it also travels round towards the South, until at noon it is directly south of us. So at noon, the tip of the shadow is due north of the nail. That is the point where the black line crosses the light blue one.
As the afternoon goes on and evening comes near, the Sun moves westwards and gets lower as it does so. So the tip of the shadow moves eastwards (to the right, in the picture) and as the Sun gets lower and the shadow gets longer, the tip of the shadow is further and further away from the centre. This being midsummer, the Sun sets rather north of west, and so the shadow ends up rather south of east: towards the bottom of the page.
The year and the seasons: Greenwich
The Sun has one other motion apart from its daily journey across the sky. It moves from north to south and back again. On December 21 it is about 23½° south of the equator, far away from Greenwich, which is why the Sun is low at that time, the shadows are long, and the days are short. On June 21 is is about 23½° north of the equator, and a lot closer to Greenwich. So then the Sun is high, the shadows are short, and the days are long. Here is a picture of the whole half-year from December to June:
 December to June at Greenwich
December to June at GreenwichJune 21 is at the bottom of the page. The Sun rises in the north-east and sets in the north-west, and at noon it is high, which is why its shadow is so close to the centre.
December 21 is at the top of the page. The Sun rises in the south-east and sets in the south-west, and at noon it is low, which is why its shadow is so far from the centre.
March 21 is a special day. On that day the Sun rises exactly in the east and sets exactly in the west. Its shadow is a straight line on that day, and it is marked by a blue line in the picture. The Sun rises at 6am and sets at 6pm. This is true not only at Greenwich but everywhere in the world apart from the North and South Poles. The day is 12 hours long and so is the night. For this reason March 21 is called the Spring Equinox (equi-nox = equal-night in Latin). Astronomers prefer to call it the “Vernal” Equinox, because for Australians and Chileans March 21 is the start of autumn, not spring – although this is a bit of a con because in Latin the word "vernalis" means "belonging to spring" anyway.
There are two ways of telling the date by the Sun, and you can see them on the diagram. One is to measure the length of the shadows (or the height of the Sun) at noon; the other is to measure the direction of sunrise or sunset. Both have been used to correct calendars in the past. Stonehenge is aligned to correspond with the sunrise on important dates. You could predict your birthday by marking where on the horizon the Sun rises on that day, and each year watching as it rises closer and closer to the important point.
You will be unlucky if you are born in late June or around Christmas because the lines are closer together then: indeed, they have probably merged to black on the picture. This is because whenever the Sun reaches its northernmost or southernmost point it slows down before changing direction. For this reason June 21 and December 21 are called the summer and winter solstices: the word means “the times when the Sun stands still”.
The red lines in the picture indicate the hours. When the shadow passes the thick red line pointing upwards in the middle of the picture, it is 12 noon. The next red line to the right is 1pm and the next one to the left is 11am: the rule is straightforward. These are the lines you would mark on your sundial so you could actually use it to tell the time.
In Part I of this story of sundials on Pluto, the red lines are merely decorative. But do notice that the lines are not straight, but curved. The curvature comes from important facts about the way the Earth spins and the way it orbits the Sun. These facts are a lot more noticeable on Pluto and I’ll go into them in detail in Part II. I would like to mention now that all the diagrams I am showing you are only covering half a year, not a whole year. If I had made a diagram cover a whole year (December to December instead of just December to June) then you would have seen that the missing half of the red line curves the other way, and the two of them together make a long thin twisted loop. The shape of the loop is different on different planets, and the one on Pluto is enormous – as we’ll see later on.
The effects of latitude: the Earth
Greenwich is in the Earth’s temperate zone, which means that in solar terms it is neither here nor there. If we go down to the equator, here is what a sundial will show:
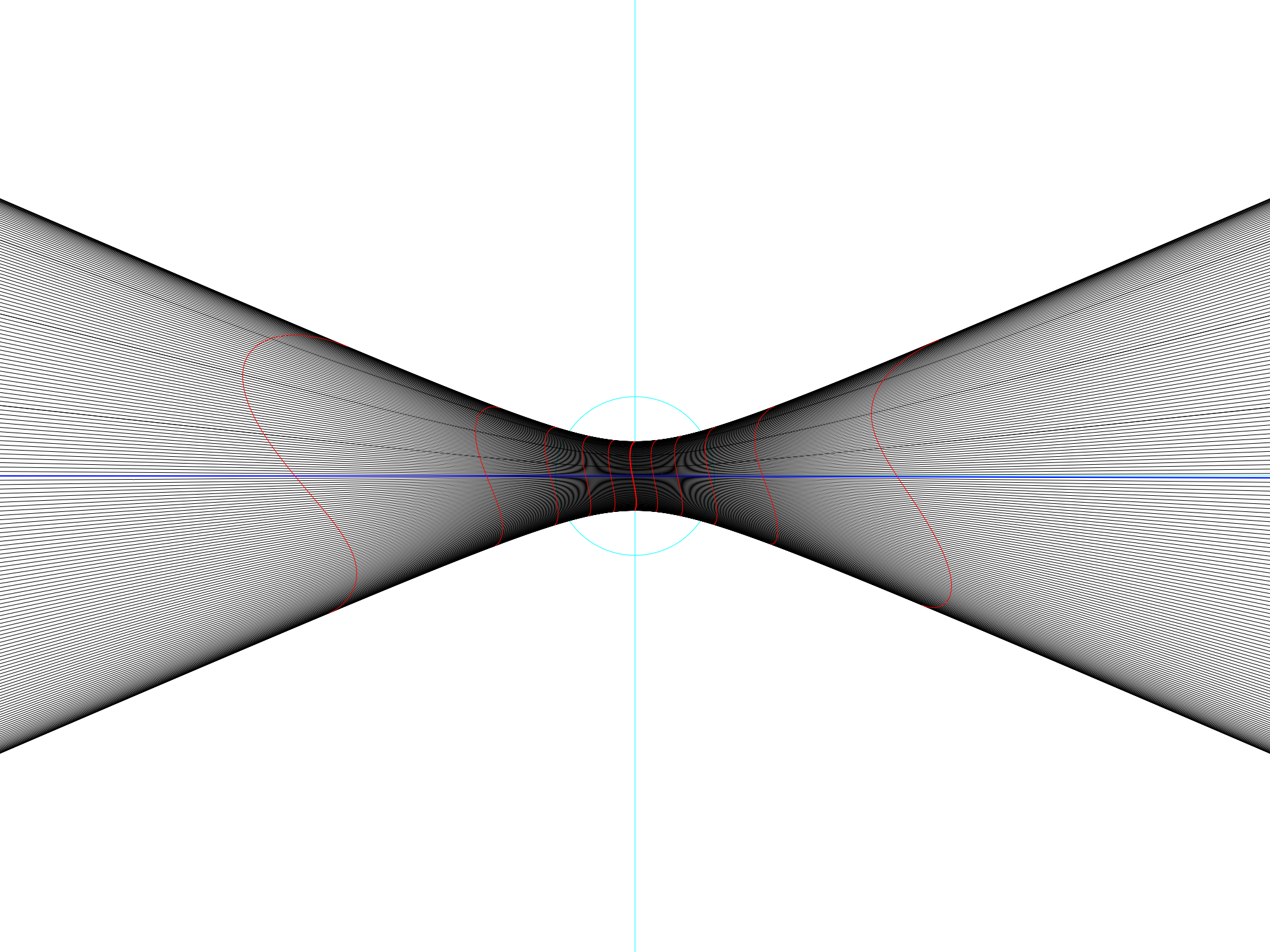 December to June on the equator
December to June on the equatorThe shadows at noon are much shorter because the Sun is much higher. At Greenwich the noon shadow was always north of the centre, but at the equator it is north and south of the centre, passing directly overhead at the equinox.
The places between 23½°N and 23½°S are called the tropics. They are the places where the Sun is directly overhead at noon on one or two days of the year. Their diagrams aren’t interestingly different, so I won’t show them, but one place at 23½°N has an important place in history because it held the key to measuring the circumference of the Earth. In the 3rd century BC, merchants visiting Syene in Egypt reported that at noon on Midsummer’s Day the Sun shone straight down a vertical well: this is because Syene was at latitude 23½°N. But Eratosthenes of Alexandria, on the north coast of Egypt, measured the length of a shadow at noon on Midsummer’s Day and found that the Sun was not vertical but, he estimated, a fiftieth of a circle away (we’d call it 7.2°). So Alexandria was a fiftieth of a circle away from Syene, so if he could only find out what distance Alexandria was from Syene, he could multiply by 50 and get the circumference of the Earth. He knew that camel caravans took 100 days to go between Alexandria and Syene, so all he had to do was ask an expert how fast an average camel caravan went… Even with rough measurements, and guesses about the speed of camels, Eratosthenes came up with a circumference of Earth within about 10% of our modern measurements.
The tropics aren’t very interesting where sundials are concerned: their diagrams look much like the one for the equator, with just a slight shift. So let’s go north from Greenwich instead, to Reykjavik in Iceland (64.13 degrees north):
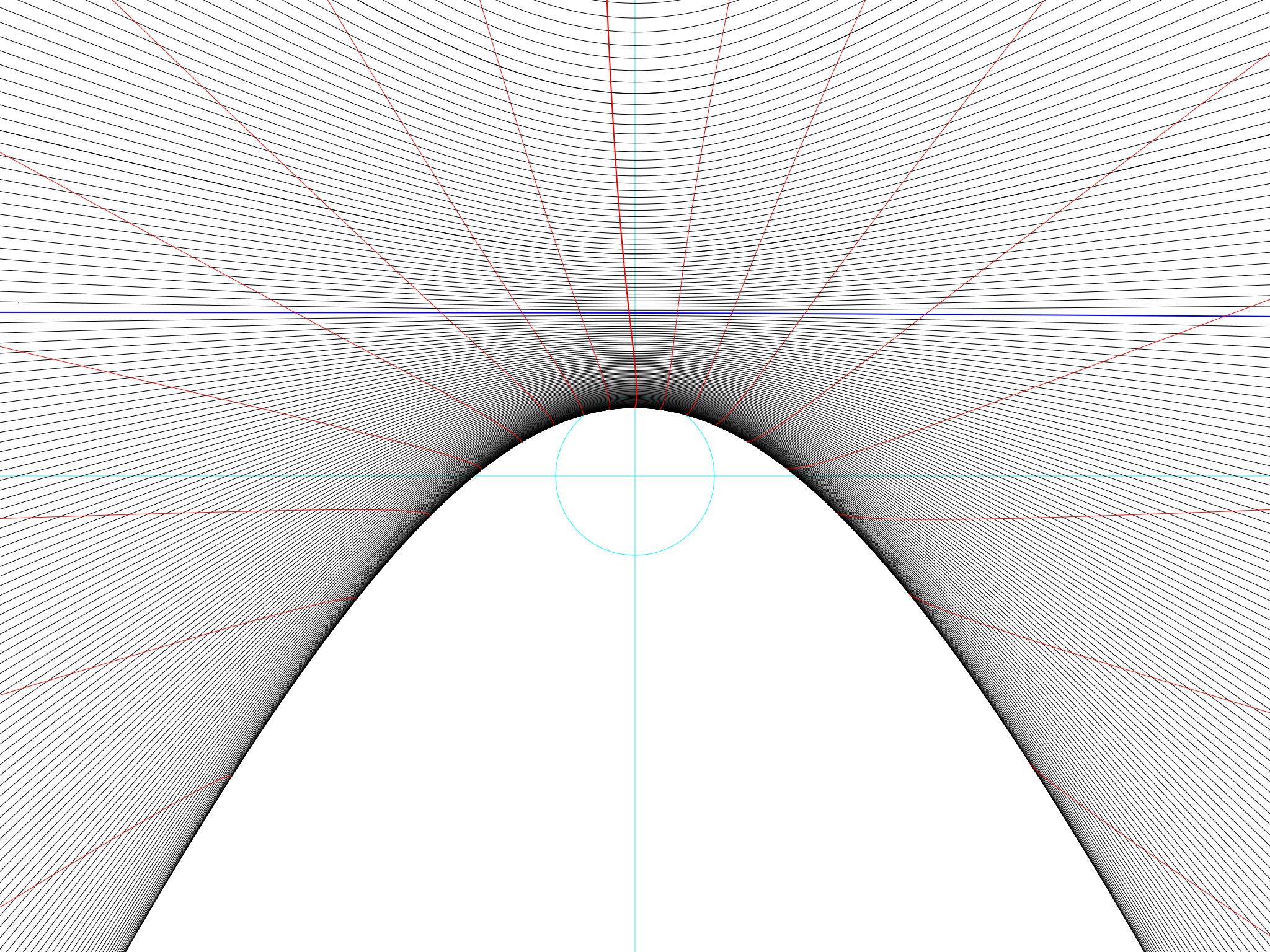 December to June, at Reykjavik
December to June, at ReykjavikThis is like the shape of Greenwich but more so. The winter Sun is so low that a lot of the shadow lines have disappeared off the top edge of the diagram; and in summer, the Sun seems to be trying harder and harder to rise in the north instead of the east. If you count the hour lines, you'll see that the Sun rises well before 4am. You might think that if this carries on, the Sun will rise in the north, at midnight, and there will be no night at all…
One step further, to Thule in northern Greenland (76.53 degrees North):
 December to June, at Thule
December to June, at ThuleIt has happened! The lines have curled round and joined together. At midsummer, the Sun never sets: it just goes round and round. At midnight the Sun shines straight from the north (quite low, casting a long shadow southwards); at noon it shines straight from the south, rather higher, casting a shorter shadow northwards. A sundial in Thule is a beautiful, circular thing. On the other hand, the Sun in winter is so low that its shadows are too long for the diagram, and for a few weeks it circles benerath the horizon and never rises at all.
Finally, at the North Pole (90 degrees North), the victory of the circles is complete:
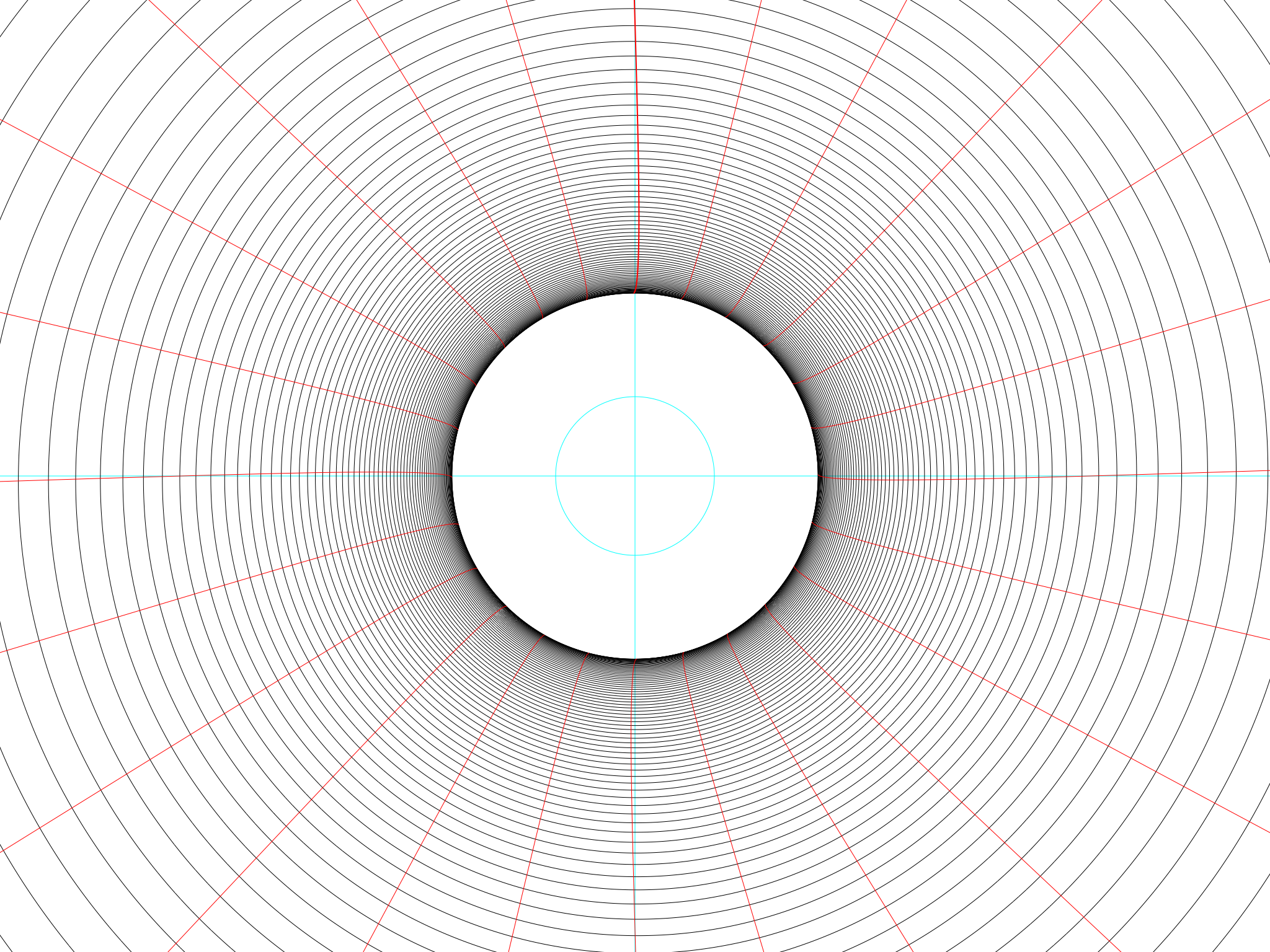 March to June at the North Pole
March to June at the North PoleIn the whole of this diagram, the Sun never rises or sets at all, it just goes round and round. If you have the patience and the eyesight then you will be able to follow its path and see that it is not a set of concentric circles but one long spiral line.
There is no blue equinox line in this diagram, because at the spring equinox the Sun is spiralling up from below the horizon and taking days to do the amount of rising that in the tropics would take less than a minute. You can say, if you like, that the equinox line is there, but infinitely far away.
And that is the end of our journey on Earth. Now it is Pluto’s turn.
The sundial on Pluto
First of all, I have to warn you that I am going to cheat. There are 182 Earth days in half an Earth year, so I could afford to draw them all. A Pluto year is almost 250 Earth years long and a day on Pluto is over six Earth days, and putting the figures together, a year on Pluto is about 14,139 Pluto days long. There is no way to draw a diagram with fourteen thousand lines on it – it would all be black – so I will only show every 50th line or so instead of every single one. Here is what the sundial will show at Pluto’s North Pole:
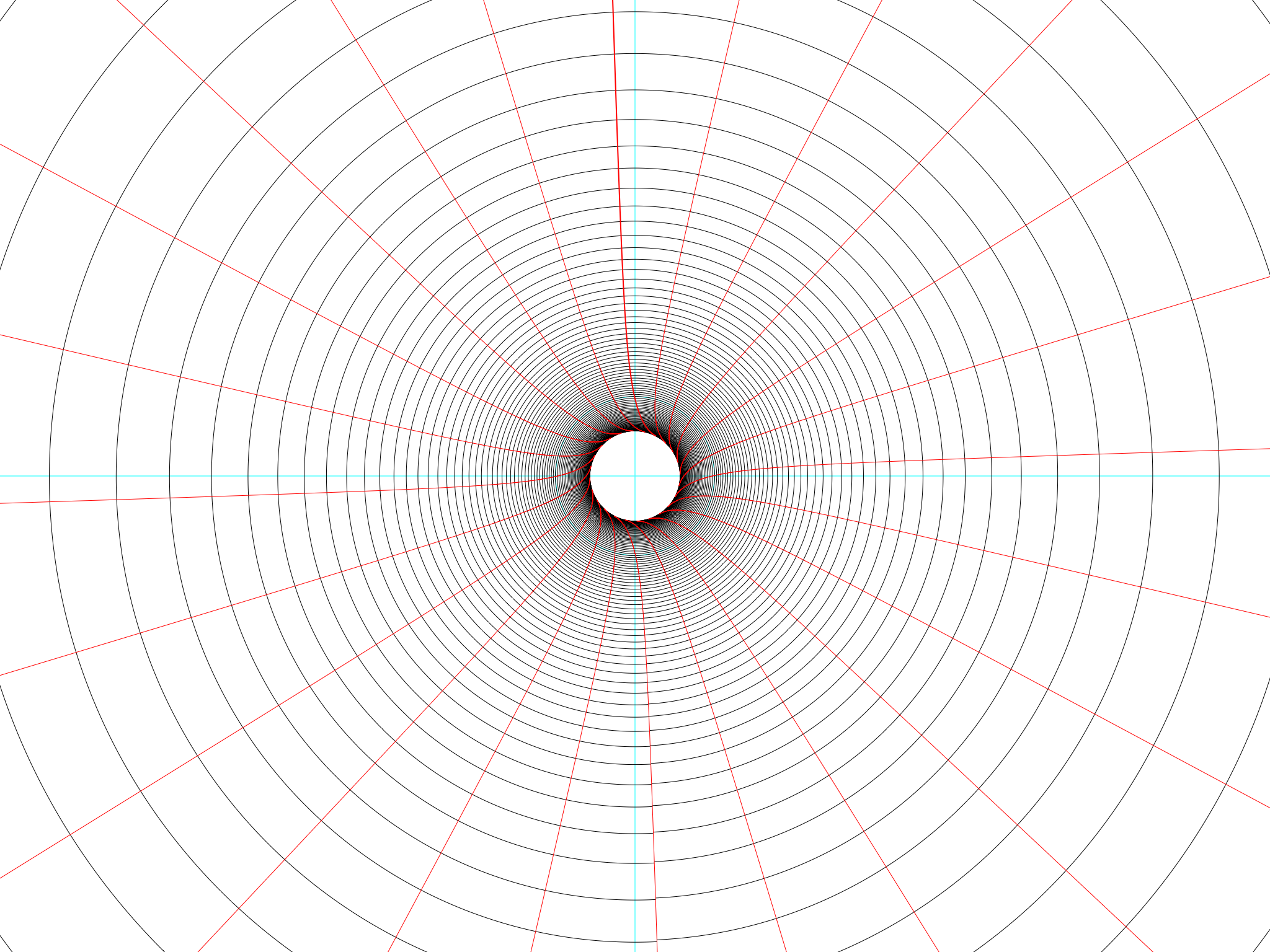 A half-year at the north pole of Pluto
A half-year at the north pole of PlutoIt really looks much like the Earth’s North Pole. The circle is smaller because at midsummer on Pluto, the Sun at the North Pole is much higher than it is on Earth.
Down at Pluto’s equator:
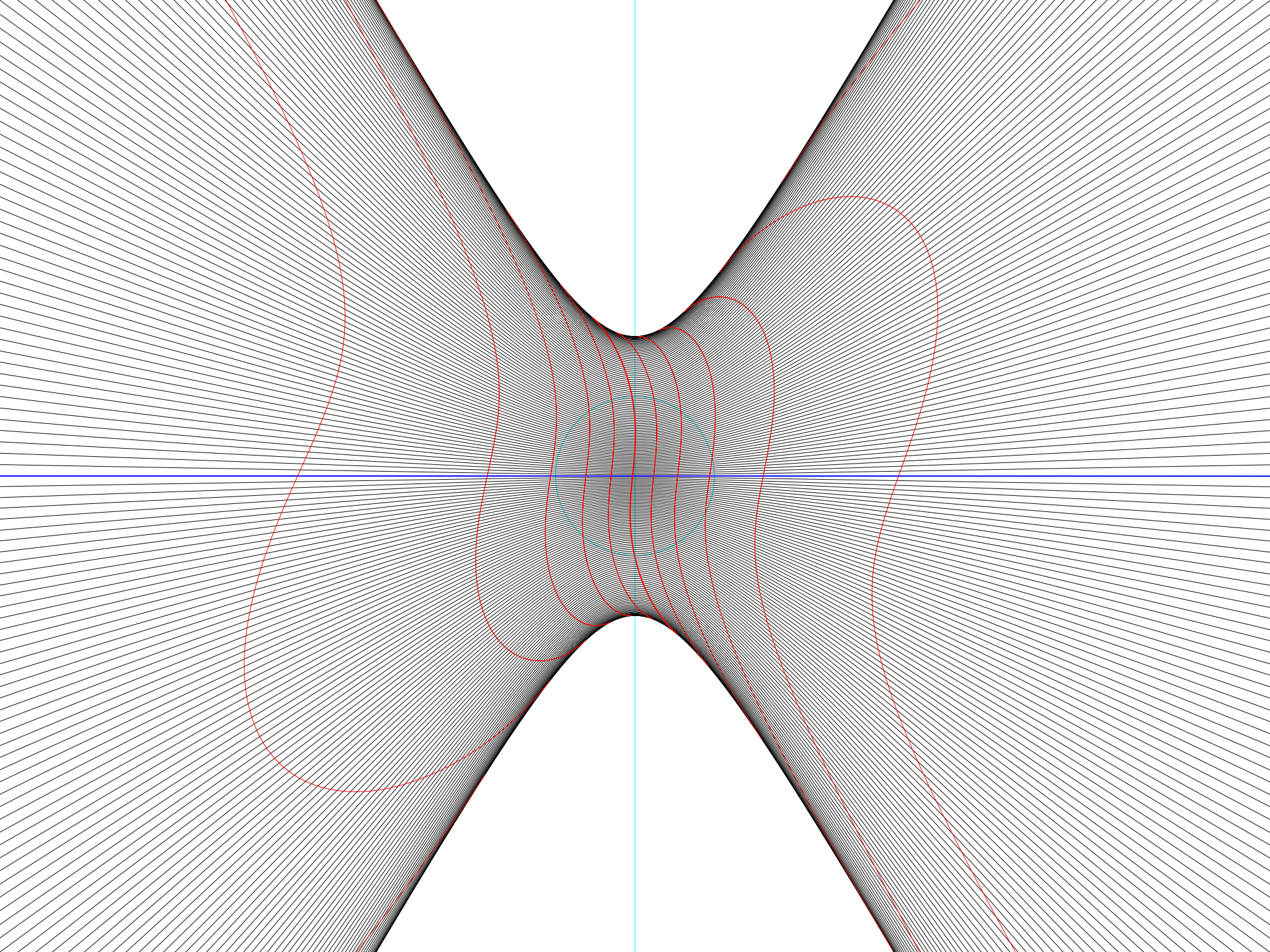 A half-year on the equator on Pluto
A half-year on the equator on PlutoThe shadows do look sort of equatorial, in that they are a symmetrical ribbon passing over the centre of the diagram. Undoubtedly, if you stand on Pluto’s equator then twice a year the Sun will be directly overhead at noon, just as it would be on the Earth.
But if Earth’s equator made a long thin ribbon, Pluto’s is making a much fatter one. In summer the Sun is trying to rise in the far north and in winter it is trying to rise in the far south – just as if, on Pluto, the equator were trying to do its best to be Reykjavik.
But look at those red hour lines. They twist around amazingly. It looks as if noon is sometimes at 9pm or 3am, Pluto time, and on some days the Sun rises when your watch says the time is 12.
I’ll go into this more in Part II, because it’s one of the most interesting facts about Pluto and it should make us feel lucky that we live here and not there. The thing to remember for now is that the hour lines show the time that would be indicated on a smoothly and evenly ticking clock. On Earth, this is reasonably close to what the Sun actually does, but on Pluto it isn’t. The first astronauts to visit Pluto will have to think carefully about how to set their watches.
A look at 51.48 degrees North (the longitude of Greenwich, on Earth) reveals the true strangeness of Pluto:
 A half-year at 51°N on Pluto
A half-year at 51°N on PlutoOn the Earth, Greenwich is neither one extreme nor the other. It isn’t in the Arctic Circle, where the Sun goes round and round for days without ever setting and then, in winter, never rises at all for weeks at a time. It isn’t in the tropics either, where the Sun is directly overhead at noon.
Greenwich-on-Pluto is both these things at once. It is both in the Arctic Circle and in the tropics. Look at the diagram. At midsummer the Sun goes round and round the sky and never sets (which means, though we obviously can’t see it here, that in midwinter it spends a long time never rising). That is Arctic behaviour.
But at the same time, even while the Sun is going round and round, it tropically shines from directly overhead. To see this, look carefully at the diagram: the central light blue circle is partly covered by the Sun’s path, and the point where the light blue lines cross (when the shadow’s length because the Sun is overhead) has shadow lines going straight over it.
Pluto: the Arctic tropics
The first big difference between Pluto and the Earth is easily explained. The seasons happen because the Earth’s axis is tilted relative to the sun. The North Pole is tilted 23½° towards it in June, 23½° away from it in December, and parallel to it in March and September. That gives us summer and winter and spring and autumn, and it also gives us the tropics (within 23½° of the equator) and the Arctic and Antarctic Circles (within 23½° of the North and South Poles).
Pluto is also tilted, but differently. Relative to its orbit, its tilt is not 23½° but 60½°. (Its orbit is also steeply tilted relative to the Earth’s orbit, but that is only important to rocket scientists). So here, in a table, is how the zones work on the Earth and on Pluto:
| Earth | ||
|---|---|---|
| Within 23½° of the equator | 0 – 23½°N | Tropics |
| Within 23½° of the North Pole | 66½° – 90°N | Arctic Circle |
| In the middle | 23½° – 66½°N | Neither tropics nor Arctic Circle: the temperate zone |
| Pluto | ||
| Within 60½° of the equator | 0 – 60½°N | Tropics |
| Within 60½° of the North Pole | 29½° – 90°N | Arctic Circle |
| In the middle | 29½° – 60½°N | Both tropics and Arctic Circle |
Will watches tell the time on Pluto?
We now know how a sundial will work on Pluto, and, as a bonus, how its strange climatic zones are arranged. But there are still those crazy hour lines to worry about. At the equator on Pluto they take on extreme shapes:
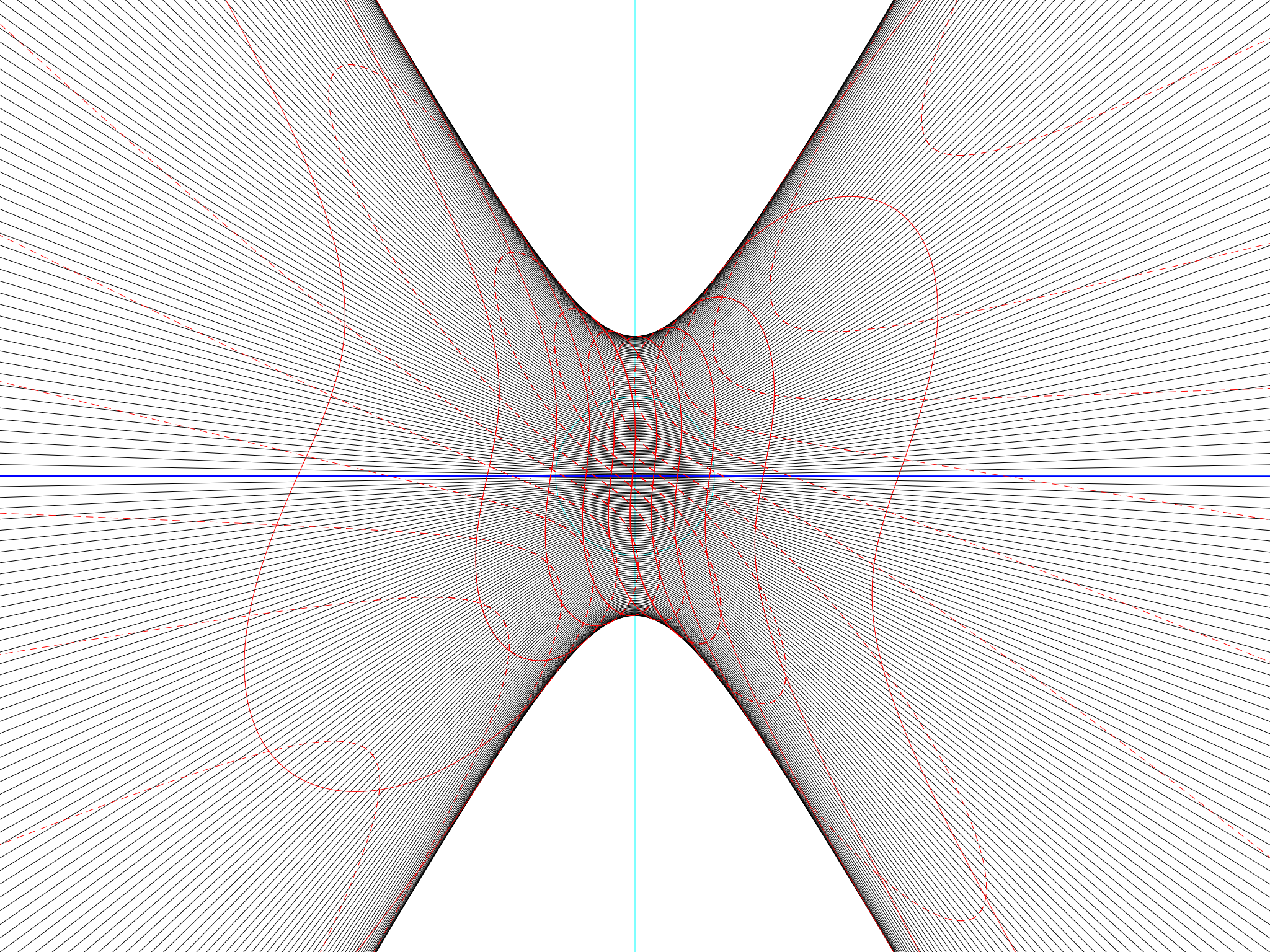 A full year at the equator on Pluto
A full year at the equator on PlutoThe solid red lines (between December and June) are snake-like but at least they are approximately vertical. The dotted lines represent June to December, and they are all sloping off at a steep angle as if they belonged to another planet altogether.
The question is a big one. On Earth, there is sundial time, working from the position of the Sun, and there is clock time, working from the position of a completely imaginary “mean” or average Sun. Most of us don’t have any reason to reflect on the difference, and on whether the difference makes any difference. On Pluto, those writhing red lines show that there is a big difference, possibly even big enough to make watches pointless.
Part II will delve into the cause of these shapes and why they are so different on Pluto – and what, if anything, we could do about it if we ever went there.